Workshop

Harmonic and anharmonic oscillations

studied with CBL and Graphic Calculators

Giacomo Torzo

INFM, CNR and Physics Dept. of Padova University

Giorgio Delfitto

Physics Dept. of Padova University

Barbara Pecori

Physics Dept. of Bologna University
 
 
 
 
 
 
 
 

Mechanic oscillating systems on-line

Using on-line apparatuses (sensors + interface + calculator) to study oscillatory motions may be very useful in the physics laboratory because the system evolutionin time becomes directly accessible.

Plots of position, velocity and acceleration vs. time allow an immediate evaluation of the essential characteristics of the observed motion.

Also the experimental investigation within less common graphic representations (velocity/position, acceleration/position, force/acceleration, force/position …) may offer precious hints for a deeper understanding of the studied phenomena.

To illustrate the potentialities the on-line experiments, we will show several oscillating systems: some of them are normally part of introductory courses in mechanics, while others are less commonly performed because they are strongly anharmonic.

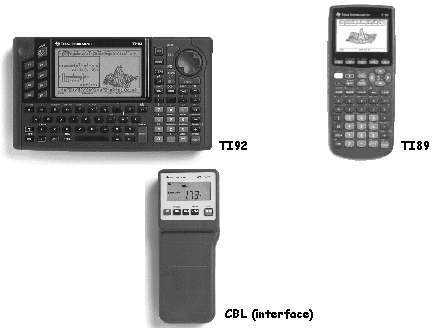
Using this simple & cheap on-line apparatus, many experiments on oscillations can be easily performed : Mass-spring oscillator, Pendulum, Atwood oscillator, Bouncing ball, Cart on incline, Galileo oscillator, Yo-yo (or Maxwell wheel), See-Saw on round or square pivot…

The majority of these phenomena are intrinsically anharmonic motions, as most of the ordinary-life mechanical oscillations. This is due to the fact that usually the restoring force is produced by some component of the gravity field, which is constant.

In some cases a quasi-harmonic motion may be obtained by choosing a proper system geometry (pendulum) and small departures from equilibrium position.

Intrinsically harmonic motion is produced only by a restoring force which is linearly dependent on the displacement, as in the case of mass-spring oscillator, or of the Atwood oscillator (when the immersed body has uniform cross section).