Rüchardt's experiment
for measuring γ=cp/cv in air

The
apparatus (figure 1) allows to measure the ratio γ=Cp/Cv
for air, using a modern version of the Rüchardt method, where Cp=specific
heat at constant pressure and Cv=specific
heat at constant volume.
With this apparatus one simultaneously measures the changes of three thermodynamic variables (pressure, volume and temperature) during the fast volume oscillations induced in a mass of air by a low-friction sliding piston (adiabatic transformation).
Volume is measured by a motion sensor (sonar), pressure is measured by a pressure sensor (barometer), temperature is measured by a special sensor that monitors the resistance of a tungsten wire .
With this apparatus one simultaneously measures the changes of three thermodynamic variables (pressure, volume and temperature) during the fast volume oscillations induced in a mass of air by a low-friction sliding piston (adiabatic transformation).
Volume is measured by a motion sensor (sonar), pressure is measured by a pressure sensor (barometer), temperature is measured by a special sensor that monitors the resistance of a tungsten wire .
In
adiabatic regime, where the Poisson relation holds (PVγ=cost),
the γ value may be obtained not only, as in classic Rüchardt
method, from the measured
oscillation period, but also from
various P-V-T plots.
For small changes in pressure dP and volume dV the Poisson relation is dP= -(γP/V)dV
A small displacement x of the piston from the equilibrium position, produces a volume change dV = xA (where A is the piston cross section) and a restoring force :
For small changes in pressure dP and volume dV the Poisson relation is dP= -(γP/V)dV
A small displacement x of the piston from the equilibrium position, produces a volume change dV = xA (where A is the piston cross section) and a restoring force :
F
=A dP = - A2 x (γ P/V).
The free piston oscillations describes therefore a (damped) harmonic motion (figure 2).
A plot of the reduced variables dP/P0 vs. dV/V0 (figure 3) is a straight line with slope - γ.
By substituting P=nRT/V into the Poisson equation we get the relation TV(γ-1) = const, from which :
dT/T=
(γ-1)
dV/V
which allows to evaluate γ
from a plot of dT/T
vs. dV/V : in figure 4, the graph shows a
Lissajous curve approximating an elliptical spiral due to
the time-lag in the thermometer response. Moreover, in
figure 4 the nominal thermometer output was corrected by a
factor 1.38, to obtain the expected slope value (-0.4),
accounting for the attenuation of the signal due to the
intrinsic low-pass filter provided by the thermometer finite
heat-capacity.
The dynamic may be varied by changing the value of the oscillating mass m (by loading the piston with metal rings) or the effective air volume V (by inserting ping-pong balls into the vessel ).
see: A new MBLversion of the Rüchardt experiment Am. J. Phys. 69, (2001)
The dynamic may be varied by changing the value of the oscillating mass m (by loading the piston with metal rings) or the effective air volume V (by inserting ping-pong balls into the vessel ).
see: A new MBLversion of the Rüchardt experiment Am. J. Phys. 69, (2001)

Figure 1

Figure 2

Figure 3
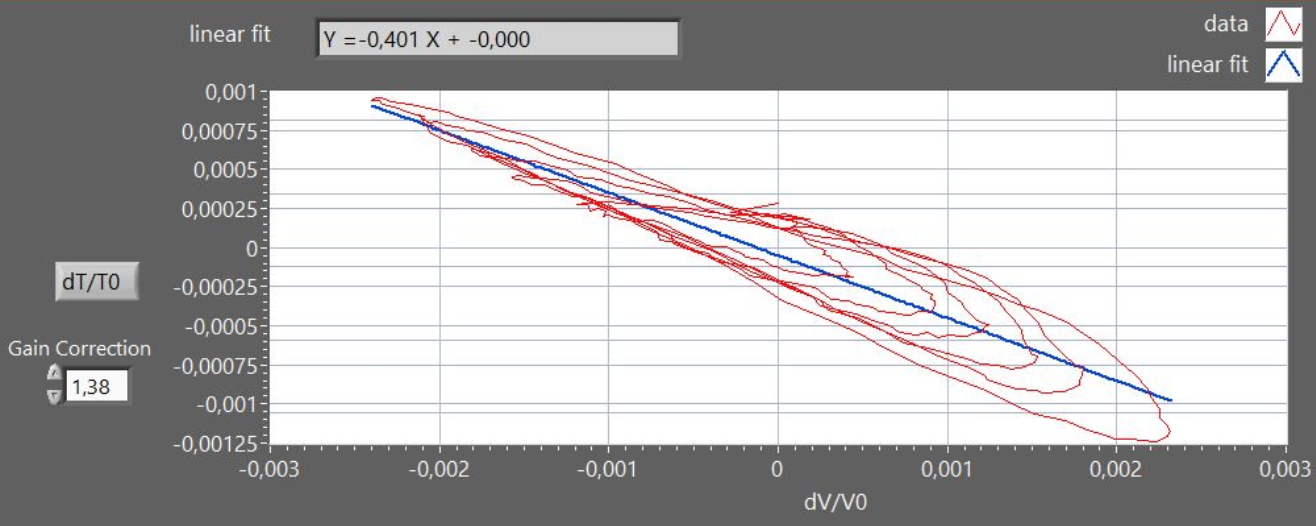
Figure 4